Here's the problem:

Consider the area beneath y = 1/x, from x = 1 to x = L, revolved about the x-axis. The volume is: Now consider the area under the curve. It's:

Now we let L → ∞ and note that ...

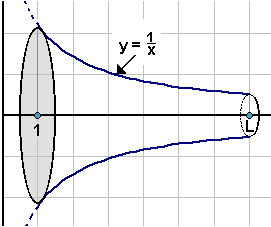>The volume becomes π, right?
Yes, but what about the area? In fact, A → ∞

We're left with the conclusion that we can have a container, like this filled with no more than π cubic metres of paint ... no matter how deep the container ... yet there's apparently not enough paint to cover the cross-sectional area. >Huh? Don't you see? It's a kind of paradox. The area A becomes infinite, but the volume doesn't!

>That's never happened to me. When I paint something ...
Okay, here's your problem. We'll talk about real paint, not mathematical paint.
If the molecules of paint have radius r, you can only fill the container until the radius of the container is r.
Further, if you paint the area 2A one molecule thick, show that there's no paradox.
Indeed, there will always be enough paint in the container to cover that area.

>That's my problem?
Yes.

>zzzZZZ

Okay. If we fill the container until y = 1/x = r, then the paint lies between x = 1 and x = L = 1/r. The volume of paint (from x = 1 to x = 1/r) is V = π (1 - r). Our crossectional area (from x = 1 to x = 1/r) is 2A = 2 ln(1/r). The volume of paint needed to cover the crosssectional area to a thickness r is: v = 2A r = 2 r ln(1/r). Would you be surprised to know that v = 2r ln(1/r) is always less than V = π (1 - r) when r is small? Or, perhaps it's better to say that 2 ln(L) / L is always less than π (1 - 1/L) when L is greater than 1. Would you like to tackle that problem? >zzzZZZ