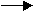Once upon a time (before I retired) I enjoyed teaching introductory calculus.
Like many (most?) other teachers, I would introduce Lebniz's notation dy/dx for the derivative, noting that this expression was not a quotient, but a convenient symbol for the derivative, indicative of its origin (as the limiting value of Δy/Δx).

Then, at some point, I would actually define the differential dy, like so:

If y = f(x) then dy = f '(x) dx >Huh? Did dy and dx now have a life of their own? Yes, indeed. If y = x2 then, according to our definition, dy = 2x dx and now dy is a function of both x and dx. Although I'd call dx and dy infinitesimals, I might set dx = 2 !. If, for example, x = 3 and dx = 2 then dy = 2(3)(2) = 12, as shown here If we choose a small value for dx, then the values of dy and Δy are nearly equal. >And your students were confused, right? Indeed. In fact I'd sometimes write the definition as: dy = (dy/dx) dx since dy/dx was just another name for f '(x) ... and it seemed I was just cancelling out the dxs. But they were even more confused when I tried to find the function y = f(x) such that dy/dx = y2.

the differential

I'd rewrite the differential equation as (1/y²) dy = dx and try to find u(y) such that du = (1/y²) dy.
Since du = u'(y) dy (as per definition of the differential), then u'(y) = 1/y² and students could easily find such a u(y), namely u(y) = -1/y.
Our "rewritten" differential equation then became: du = dx   so   u = x + C or -1/y = x + C and that was the solution.

>And your students were no longer confused?
Are you kidding? I'd play with these differentials like crazy, adding and subtracting them and multiplying variables by differentials and ...

>Now I'm confused!
I'd write r² = x² + y² and take differentials of everything in sight, writing:   2r dr = 2x dx + 2y dy.

Then, later in the course I'd write things like: 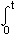  f(x) dx   where, again, I'd refer to "dx" as an infinitesimal.

>So do you ever justify all that differential manipulation? Is it a number? Is it an infinitesimal? Is it ...?
No, I just remind them that it works ... and there are rules for manipulating these magic creatures called differentials, such as:

• d(u + v) = du + dv
• d (uv) = u dv + v du
• d (u / v) = (v du - u dv) / v²
• If u = f(y) and y = g(x) then du = f '(y) dy and dy = g'(x) dx so du = f '(y) g '(x) dx

That reminds me of Heaviside Calculus.
Oliver Heaviside (1850-1925) was a self-educated British physicist. He solved difficult problems by introducing an "operator" which I'll call D.
It stood for the derivative, so that he'd write Dy in place of dy/dx.
Then, since D [e^ax] = a e^ax, the result was obtained simply by replacing D by a.
For example, if y = e^ax, then d²y/dx² - 2 dy/dx + 3 = (D² - 2 D + 3) e^ax = (a² - 2 a + 3) e^ax ... replacing D by a.

Further, D[ f(x) e^ax ] = e^ax (D f(x) + a f(x)) via the Product Rule.
So he'd write:   D[ f(x) e^ax ] = e^ax (D + a ) f(x)
and you could just Shift the Exponential to the left, past the big D, and replace D by D + a.

And (would you believe?)   D²[ f(x) e^ax ] = e^ax (D + a )² f(x) = e^ax (D² + 2a D + a²) f(x).

>I assume that D² means the 2nd derivative.
Yes, and D^-1 means - you guessed it - the integral!

If you wanted to integrate x² e^ax, you'd write:

Dy = x² e^ax so y = D^-1 [ x² e^ax ] = e^ax (D + a)^-1 [ x² ] ... Shifting the Exponential and replacing D by D+a
so y = (1/a) e^ax (1 + D/a)^-1 [ x² ] = (1/a) e^ax (1 - D/a + D² / a² - ... ) x² ... using a power series expansion
so y = (1/a) e^ax ( x² - 2x / a + 2/a²) ... since higher derivatives of x² are zero.

>And Heaviside got away with that?
He was severely criticized, but no one could deny that his solutions to problems were correct.
If you want, you can differentiate (1/a) e^ax ( x² - 2x / a + 2/a²) to see if you get x² e^ax.

>No thanks. But can't one show that Heaviside Calculus is valid? I mean ...
Eventually, as one might expect, Heaviside Calculus was given the stamp of approval ... and justified using Lapace Transforms.

>So were your students happy with manipulation of those differentials?
Actually, after a time they had little difficulty dealing with and manipulating dx and dy, just as students in a later course on Differential Equations has little difficulty in using Heaviside Calculus to find a solution. If I gave them
        y'' - 2y' - y = x e^x
they'd immediately write:
        y = (D² - 2D - 1)^-1 [ x e^x]
then that shift and long division (or a power series expansion) and ...

>Yeah, I get it. I reckon you were more concerned 'bout "what's a differential?" than your students.
Uh ... yes. In fact, my students were never really concerned with the justification for using differentials ... they just used them!
Eventually, however, an infinitesimal calculus was developed called nonstandard analysis that gave validation to working with things like dx and dy.

>But can't you avoid talking about differentials?
Yes, but if students do Science or Engineering as well as higher level math courses, they'll see them again and again.
Indeed, they'll run across stuff like:

• 
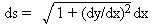
• dr² = c²dt² - dx² - dy² - dz²
• _∫∫ f(x,y) dx dy
• dP = μ(t,P)dt + σ(t,P) df(t)
• 
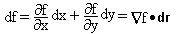
• dW = Fdr   and   dν = vdA
• ...

See Math Stuff