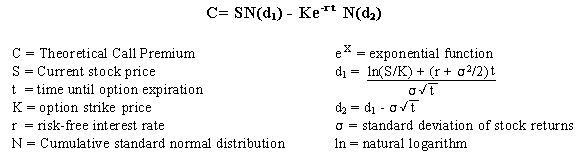So what's a Call option worth - and what does its price depend upon? If you buy a Call with a strike price of $45, you can then buy the stock for $45 per share. If the stock happens to be trading for $52, then you're going to pay at least $7 for the option; that's $7 = $52 - $45*. If the option were selling for less, say $6, then EVERYBUDDY would run out, buy the Call for $6, exercise it, buying the stock for $45 ... they've paid $6 + $45 = $51 so far ... then immediately sell the stock for $52 (the current trading price) for an instant $1 profit (less commissions, of course). So, our Call will cost at least $7. Hence, the Call option price (or "premium") will undoubtedly depend upon the Stock Price and the Strike Price.
* Remember this formula! Aah, now suppose the expiry date is months away. The stock could very well be worth considerably more than $52 by then ... so, unless the expiry date is tomorrow (or sooner!), you're going to pay MORE than that $7. How much more? It depends upon the eventual price of the stock, umpteen days into the future ... and that, of course, depends upon the number of Days to Expiry and the Volatility of the stock. So far, we've got the option premium depending upon four things, but there's more. If we go through some elaborate calculation and determine that the "expected" Option Premium will be $X at expiration, then what's the option worth now? Something that's worth $X in umpteen days will be worth the Present Value of $X right now. This Present Value will depend upon some interest rate ... a Risk Free Rate. Now we have the option price depending upon five things.
Okay, it's time for the magic Black-Scholes option-pricing formula:
However, at expiry, t = 0, e-rt = 1, d1 = d2 = infinity so N(d1) = N(d2) = 1 and we'll get, again, C = S - K.
The second term computes the Present Value (that's what the term e-rt does) of the "expected" exercise price at expiration.
The use of the word "expected" naturally implies some kind of probability distribution, and
that's
given by the normal distribution terms, N(d1) and N(d2). Personally, I think there are too many variables ... so if we express all the dollar values, like Stock Price (S) and Call Premium (C) as a multiple of the Strike Price (K) ... by dividing the Black-Scholes formula by K, we get something neat:
For example, we can now generate charts without knowing the Strike Price, but expressing
dollar values as multiples of a Strike Price. So, does Black-Scholes do a good job of estimating actual call option premiums? (By actual, I mean the price that the option actually sold for ... in the matket.) I followed Ford options, strike = $25, expiry in Oct, 2000, starting on Aug 31, 2000 and it looks like the following chart (where I invented a Volatility and Risk-free Rate in order to get a reasonable match between the actual and estimated premiums ... that's an implied volatility & rate). Oh, I forgot to mention: if y'all wanted to compute the volatility, head over to Standard Deviation stuff and learn how.
 Did Black-Scholes do good? Of course, if everybuddy uses Black-Scholes to make decisions regarding options, it sorta guarantees that Black-Scholes will do good, eh? Oh, one other thing: in the above chart, I used the closing prices of the stock and the last option trade of the day, but they didn't necessarily occur at the same time (which may account for some of the discrepancies between the Black-Scholes Estimate and the Actual Call Premium).
Enough of the mathematical details - no need to understand them fully (I don't). Remember, it was worth a Nobel Prize, so you wouldn't expect it to be simple! It's more important to know how each of the five variables affect the Option Premium ... so here are some pretty pictures, each showing the variation of the Black-Scholes Option Price when just one of the five variables changes.
 Fig. 1: Dependence upon Stock Price Options way out-of-the-money are cheep, cheep. Who wants an option with strike = $50 when the stock is trading at $35?
Note the dependence of delta upon the current stock price:
 For example, if the stock price is about 5% above the strike price, then delta is about 0.8 (where we're assuming 25% volatility and 6% risk-free rate). Just in case y'all wanna stick this stuff into a spreadsheet:
Oh ... by the way, there's a significant difference in the "Cumulative Probabilities" (which run from a probability of 0.00 to a probability of 1.00), namely N(d1) and N(d2), for in-the-money and out-of-the-money call options:
 in-the-money Note: delta = N(d1) is nearly 1
Suppose we bought a call option for $9.80 with a Strike Price of $30. At the time we bought the option the stock was selling for $39 and there were 150 days to expiry. Now, here's a neat chart:  Fig. 6
When we bought the call we had (Stock Price)/(Strike Price) = $39/$30 = 1.3 or
130%. Big Question: How much will our option be worth when there are 75 days to expiry? And what if the stock has jumped to $45 by then?
Now let's see. When the stock is at $45, that's $45/$30 = 1.5 or
150% of the Strike Price. Of course, the graphs depend upon the Volatility and Risk-free Rate ... so we've picked a couple of numbers just to give y'all the general idea. One last thing: notice that, as the number of days left (to expiry) approaches zero, the curves approach a straight line through (100%,0%), with slope "1". That means that when the Stock Price increases by 10% (of the Strike Price), the Option Price increases by 10% (of the Strike Price) ... and the "3-days to expiry" curve is almost there! Okay, one more last thing: In our example above, we bought a Call option with Strike Price of $30 and we paid $9.80 for it, and the stock itself was available on the market for $39. In order to make any money, the Stock Price had better increase to $30.00 + $9.80 = $39.80 in the next 150 days. That's a gain of $39.80/$39.00 = 1.02 or 2% (in 150 days). Is that too much to expect? It's easier to compare if this gain factor is annualized: 1.02365/150 = 1.05 so it means our stock must increase at an annual rate at least 5% (over the next 150 days) in order to make money. Now that's not asking much, eh?
So, we ask ourselves (when buying a Call option):
The result is sometimes surprising! Here's an example:
 So, is the moral to BUY deep in-the-money Calls?
Of course, if we WRITE a covered Call, we always make money unless the
Stock Price actually drops. Although that sounds better than just BUYing a Call,
our gains are limited. Then again, a deep in-the-money Call option has a large premium
and you can lose it all!
Remember:  
One-of-these-days, I'll spend a day computing, for a jillion Call options, the annualized percentage gain necessary to make money
... and consider buying the option which generates the minimum number! Here are some "required annualized gains" bumpf for some heavily traded stocks ... using the actual numbers, as of Aug. 25/00 (no Black-Scholes estimates):
See also more on Call Options. |