When I first saw the movie A Beautiful Mind I hadn't heard of John Nash. So I browsed the Net to see what won him the Nobel Prize ... a paper which Nash apparently described as his most trivial work! Anyway, I hardly understood what he had done - it was way over my head - so I forgot about it.

Then John B. pointed me to a neat PDF file and suggested it may be useful in Asset Allocation, so thought I'd try to explain ...

>I thought you didn't understand Nash?
Yeah, well, I said I'd try to explain ... that is, if I can understand the stuff.

Typically (I guess), investors use a strategy which minimizes the prospect of a "worst case" scenario. For example, the notorious 4% withdrawal rule suggests that, after retirement, one should withdraw no more than 4% (increasing with inflation) since, in the past, the worst-case-scenario had your portfolio going to zero if you withdrew more than 4%. (For example, had you retired in 1928.)

As another example, consider the following "game", played by A, B and C, each wanting to achieve some goal ... but avoiding the worst-case-scenario:

• All are in a penitentiary, on deathrow.
• They are each asked to pick a number from 1 to 1000, including numbers with decimal places (like 12.34).
• From the three picks, anyone whose pick is the middle number goes free.
• The prisoner with the largest pick gets the electric chair.
• The prisoner with the smallest pick has his sentence commuted to life imprisonment.

>I guess 0!
I assume you mean "1". So you're avoiding the worst-case-scenario, eh? But suppose ...

>Wait! What if two picks are the same or maybe they're all equal or ...
That's not important. I'm trying to make a point. Suppose ...
>The warden could start again with another game so that ...
Pay attention!

Suppose that each of A, B and C try to guess what the others will pick ... and chooses his number accordingly.
A says: I'll pick some kind of middle number. I'll pick 500
B says: A is a dummy. He's going to pick 500 thinking it's a middle number. I'll pick 499"
C says: A is no great thinker. He'll pick 500 as a middle. B is smart, going just under at 499 so I'll pick 499.5"

There's another, similar scenario called the Prisoner's Dilemma.
The Prisoner's Dilemma was invented by Al Tucker of Stanford to explain Game Theory and Nash Equilibria.
The police take two guys into custody, as suspects in a crime.
Each is interrogated separately, so neither knows what the other says.
They are each offered a deal:

• If both confess to the crime, they will each get four years.
• If neither confesses, they will each get two years ... each charged with a lesser crime.
• If one confesses and the other does not, the confessor will go free and the other gets five years.

>I confess!
To avoid getting five years, right?
However, if the other suspect thinks the same way - and confesses - you'd both get four years.
On the other hand, the outcome would be better if you both remained silent - just two years.

>Do people really think like that? I mean ...
Aah, that's where this PDF paper (mentioned above) comes in.

Consider the following game, played by thousands of people:

• Everyone is asked to submit a number from 1 to 100.
• The average of all the numbers submitted is calculated. Call it K.
• The person whose submission is closest to 2/3 of the average K wins the prize.

>It's like thinking 1 move ahead in chess, or 2 or 3 ... hey!
It'd be neat if we asked a thousand people to play that game and ...
Yes. That's what I found interesting in that PDF paper. It has been done, several times!
The game was advertised (in newspapers, for example) and over a thousand people responded to the request for numbers and there were lots of guesses at 33 and 22 and the average submission was close to 14.

>But what about people D and E and F? They'd guess lower and lower and ...
In fact, there were lots of submissions which were quite small ... less than 10. Something like the chart below.
Interesting, eh?

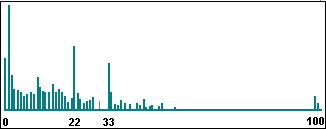
>They were the big thinkers, eh?
Maybe.
For this contest, and infinitely rational players, they'd all pick a number which is 2/3 of itself. There's only one such number; the number "0". The closest to "0", in the range 1 to 100, is "1". Yet the "best" choice, for less-than-infinitely-rational contestants, turned out to be about "14".

>And what about asset allocation?
I'm still thinking about it. In the meantime, think on this:

A Nash equilibrium is a set of strategies, one for each player, such that no player has incentive to unilaterally change his action. Players are in Nash equilibrium if a change in strategies by any one of them would lead that player to earn less than if he remained with his current strategy.

>I haven't the faintest idea what that means. I can't ...
Finding Nash equilibria seems to be a favourite pastime among economists.

Consider this: Two companies, A and B, can sell their widgets for either $1 or $2. (These are the available strategies.) The cheaper the widget price, the more will be sold. The payoff for each company is the total profit. The table shows the profit for each strategy, for each company.

Company B $1.00 $2.00 Company $1.00 $100,$100 $120,$40 A $2.00 $40,$120 $50,$50

• If each sells their widgets for $1.00, each will make a profit of $100.
  That's the cyan cell.
• If A increases its price to $2 (and B retains its strategy to sell at $1), then A will only make $40.
  That's the magenta cell.
• Similarly, if B increases its price (and A continues with its "sell at $1.00" strategy), B will make less profit.
  The orange cell.

>Uh ... any other pair of strategies ... uh ...
For any of the other three strategies a company can improve its profit by lowering its price.
The moral? Companies should stick to the Nash equilibrium since no change in strategy will improve its profit ... if other companies maintain their strategy.

>Are we still talking worst-case-scenarios?
Of course not! We're talking about how people think, how they devise strategies, how they anticipate what others will do, how they base their own strategy upon ...

>Okay! Okay!
Of course, real people may or may not adopt the Nash equilibrium.

Remember the Prisoner's Dilemma? The Nash equilibrium is, again, the cyan cell where each confesses. That way, if you change your strategy (from "confess" to "don't confess"), your sentence is worse: It goes from four years to five years. By confessing you can't do worse, no matter what the other suspect says. So, would everyone choose "confess"? And did everybuddy pick "1" for that "2/3 of the average" game? Hardly.

Suspect B confess don't confess Suspect confess 4, 4 0, 5 A don't confess 5, 0 2, 2

Our problem is to use Nash's ideas to select an investment strategy, one that anticipates what other investors may do.

>And?
I'm thinking ...

for Part II